

We write this as x ≠ 3, − 3 x \neq 3,-3 x = 3, − 3.Īfter carrying out operations on rational expressions, it should be noted that the domains of both the original and final expressions are same: they should be defined at the same input values. Multiplying and Dividing Rational Expressions We will concentrate on rational expressions with monomial numerators and denominators. Therefore, the domain of the expression is the set of real numbers, except the points 3 and -3. This gives x = − 3 o r x = 3 x=-3 \ or \ x=3 x = − 3 o r x = 3.
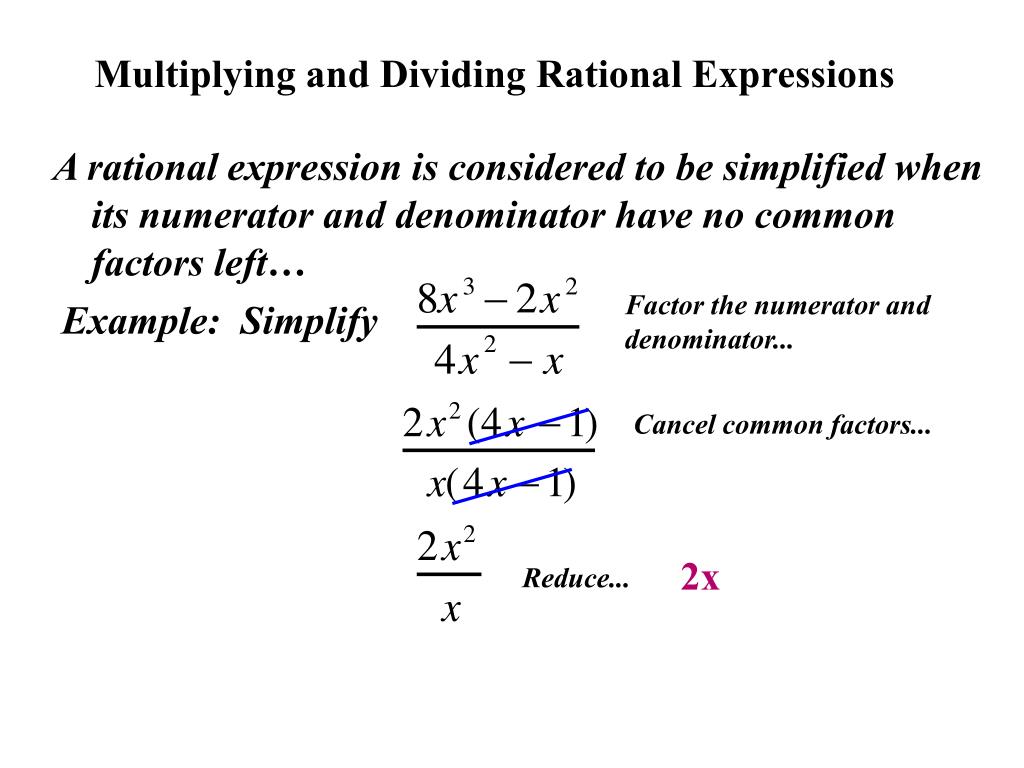
Using the property of zero product, x + 3 = 0 o r x − 3 = 0 x+3=0\ or \ x-3=0 x + 3 = 0 o r x − 3 = 0. The first fraction a b \frac ( x + 3 ) 7 ( x − 1 ) ⋅ x − 3 1 įind the zeros of the denominator and put restrictions at these points. This has been described in the panel below. Multiplying Rational Expressions Date Period Simplify each expression. When the division of fractions take place, the first fraction is multiplied by the reciprocal of the second one. Similarly, b and d are multiplied to give the final denominator. The numerators of the two fractions a and c are multiplied to give ac in the final expression. The letters a, b, c, and d indicate the polynomials.Ĭonsider the expression on top. Multiplying and dividing rational expressions works just like multiplying and dividing fractions. This is the fourth lesson in Algebra 2 Unit 8: Rational Functions. ☛ Process 3: After that a window will appear with final output.The multiplication and division of rational expressions have been described above pictorially. Color-coded and black and white-graphic organizers for simplifying, multiplying and dividing rational expressions. To multiply rational expressions, first factor all numerators and denominators and cancel any factors you can. ☛ Process 2: Click “Enter Button for Final Output”. To multiply, first find the greatest common factors of the numerator and. Now what I encourage you do is pause this video and think about what these become when you multiply them. And here, we're dividing one rational expression by another one. Before multiplying, you should first divide out any common factors to both a numerator and a denominator.
Rewrite as a rational expression and simplify when possible. ☛ Process 1: Enter the complete equation/value in the input box i.e. Rational expressions are multiplied and divided the same way numeric fractions are. Voiceover So up here, we are multiplying two rational expressions. Multiplying and Dividing Rational Expressions Multiply the numerators. Follow the given process to use this tool. This is a very simple tool for Multiplying And Dividing Rational Expressions Calculator. Easy Steps to use Multiplying And Dividing Rational Expressions Calculator Apply the Fundamental Property of Rational Numbers to eliminate the. To divide, first rewrite the division as multiplication by the reciprocal of the denominator. To multiply rational expressions:Factor all numerators and denominators as much as possible. To multiply, first find the best common factors of the numerator and denominator. Rational expressions are multiplied and divided an equivalent way numeric fractions are. What is Multiplying And Dividing Rational Expressions?
#Multiplying and dividing rational expressions free
Free Online Multiplying and Dividing Rational Expressions Calculator


 0 kommentar(er)
0 kommentar(er)
